Projectors

An operator 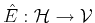$\hat{E}: {\cal{H}} \to {\cal{V}}$ is a projector if it is idempotent, i.e.,

$\displaystyle \hat{E}^2= \hat{E}.$

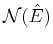
As a consequence, the projection is onto ${\cal{R}}(\hat{E})$ , the range of the operator, and along ${\cal{N}}(\hat{E})$ , the null space of the operator. Let us recall that

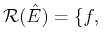
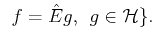
$\displaystyle {\cal{R}}(\hat{E}) =\{f, \;$ such that $\displaystyle \; f= \hat{E} g,\; g\in {\cal{H}}\}.$

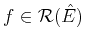
Thus, if $f \in {\cal{R}}(\hat{E})$ ,

$\displaystyle \hat{E} f = \hat{E}^2 g= \hat{E} g= f.$

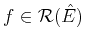
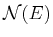
This implies that $\hat{E}$ behaves like the identity operator for all $f \in {\cal{R}}(\hat{E})$ , regardless of ${\cal{N}}(E)$ , which is defined as

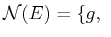
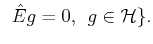
$\displaystyle {\cal{N}}(E)= \{g, \;$ such that $\displaystyle \; \hat{E} g=0, \; g\in {\cal{H}}\}.$

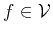
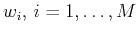
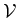
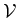
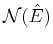
It is clear then that to reconstruct a signal $f\in {\cal{V}}$ by means of (1) the involved measurement vectors $w_i, i=1,\ldots,M$ , that we shall also called henceforth duals, should give rise to an operator of the form (2), which must be a projector onto ${\cal{V}}$ . Notice that the required operator is not unique, because there exist many projectors onto ${\cal{V}}$ having different ${\cal{N}}(\hat{E})$ . Thus, for reconstructing signals in the range of the projector its null space can be chosen arbitrarily. However, the null space becomes extremely important when the projector acts on signals outside its range. A popular projector is the orthogonal one.

Highly Nonlinear Approximations for Sparse Signal Representation

Projectors