Assume that the signal
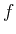
to be analyzed here is the superposition of
two signals,
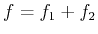
, each component being produced by a different
phenomenon we want to discriminate. Let us assume further that

and
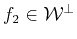
with
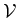
and
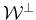
disjoint
subspaces.
Thus, we can obtain,

say, from
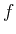
, by an oblique projector onto
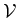
and along
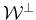
. The
projector will map to zero the component
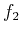
to produce