Example 4

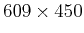
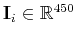
Here we deal with an image of a poster in memory of the Spanish Second Republic shown in the first picture of Fig. 2. This image is an array of $609\times 450$ pixels that we have processed row by row. Each row is a vector ${\mathbf{{I}}}_i \in \mathbb{R}^{450}$ , $i=1,\ldots,609.$ The image is affected by structured noise produced when random noise passes through a channel characterized by a given matrix

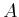
having

columns and

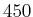
rows. The model for each row ${\mathbf{{I}}}^o_i \in \mathbb{R}^{609}$ of the noisy image is

$\displaystyle {\mathbf{{I}}}^o_i = {\mathbf{{I}}}_i + A {\mathbf{{h}}}_i,\quad i=1,\ldots,450,$

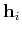
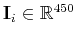
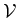
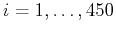
where each ${\mathbf{{h}}}_i$ is a random vector in $\mathbb{R}^{160}$ . The image plus noise is represented in the middle graph of Fig. 2. In order to denoised the image we consider that every row ${\mathbf{{I}}}_i \in \mathbb{R}^{450}$ is well represented in a subspace ${\cal{V}}$ spanned by discrete cosines. More precisely, we consider ${\mathbf{{I}}}_i\in {\cal{V}}$ for $i=1,\ldots,450$ , where

$\displaystyle {\cal{V}}={\mbox{\rm {span}}}\left\{{\mathbf{{x}}}_i=\cos\left(\frac{\pi(2j-1)(i-1)}{2L}\right),\quad j=1,\ldots,609\right\}_{i=1}^{290}.$

The space of the noise is spanned by the

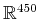
vectors in $\mathbb{R}^{450}$ corresponding to the columns of the given matrix

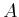
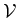
. The image, after being filtered row by row by the oblique projector onto ${\cal{V}}$ and along the space of the noise, is depicted in the last graph of Fig. 2.

**Figure 2:** Image of a poster in memory of the Spanish Second Republic (first picture). Image plus structured noise (middle picture). The image obtained from the middle picture by an oblique projection (last picture).

Highly Nonlinear Approximations for Sparse Signal Representation

Example 4